The formula a3-b3 is used to calculate differences between 2 cubes. The the formula a3-b3 is (a-b)(a2 + ab2 +). In contrast the formula for a3+b3 is A3 + B3 equals 3ab(a + b) + (a + b)3 or A3 + B3 = (a2 + ab + b2)(a + b). It is among the most crucial formulas in algebra, and this formula can be used in a wide range of areas. In this article, we’ll examine different aspects of the formula and its formula’s proof.
a3 b3 Formula Algebraic Equation
The algebraic expression, commonly called a polynomial equation is a mathematical equation in the form of:
P = 0
P is a type of polynomial that has coefficients within a specific field, often it is the area of rational numbers. A lot of authors use the term algebraic equation for univariate equations or polynomial equations having just one variable. Polynomial equations, in contrast may have multiple variables. The polynomial equation is often preferred over algebraic equations in situations where there are multiple variables (multivariate scenario). These equations comprise constant terms (1 2, 3,) and variables (x, Z, y) elevated to a certain power or a mix of both. Some instances of equations that are algebraic include 4x + 3, 7, 2×2, 4×2 + 3x.
a3 b3 Formula
A3 B3 Formulas are provided below.
(a + b)3 = a3 + b3 + 3ab (a + b)
(a – b)3 = a3 – b3 – 3ab (a-b)
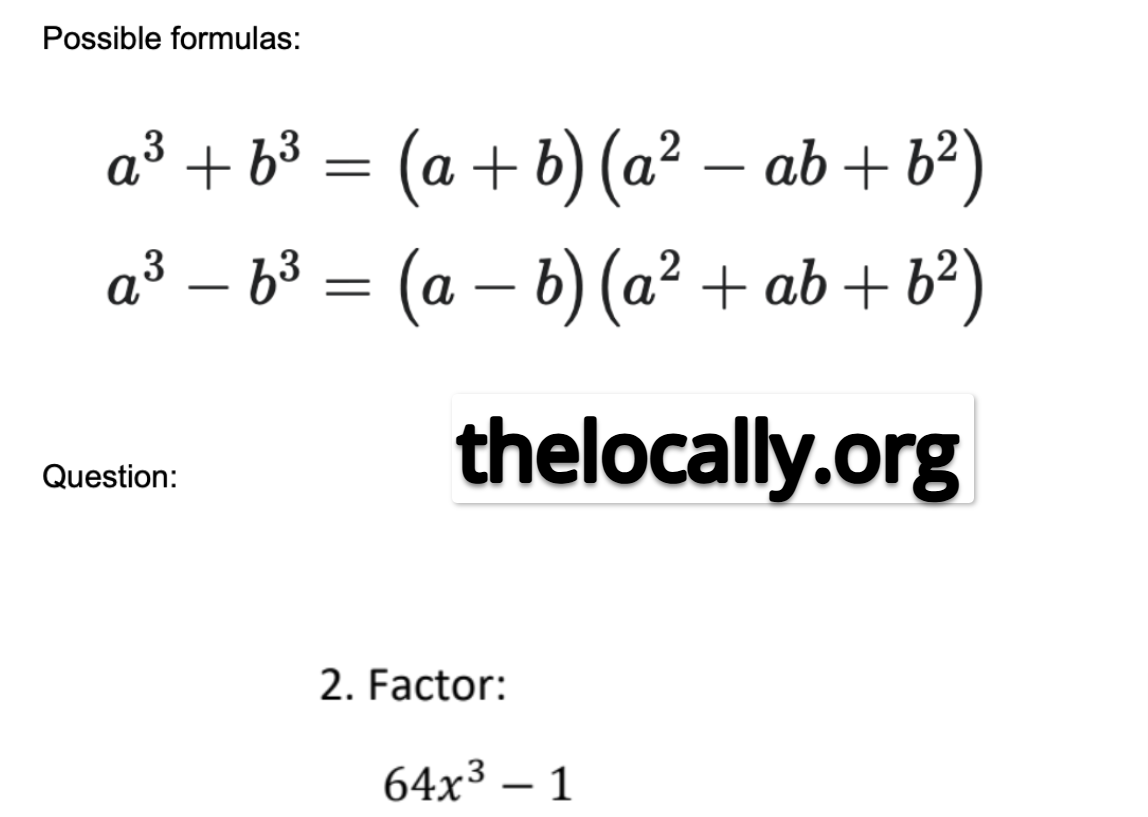
a3 + b3 = (a + b) (a2 – ab + b2)
a3 – b3 = (a – b) (a2 + ab + b2)
a3 + b3 + c3 – 3abc = (a + b + c) (a2 + b2 + c2 – ab – bc – ca)
a + b = (a3 + b3 ) / (a2 – ab + b2)
A3-b3 Formula – a^3 – b^3 Formula
The formula you’re using, “a3 – b3,” symbolizes the difference between cubes. The formula is a mathematical identitiy that can be incorporated into binomials as a product. The formula is like this:
a^3 – b^3 = (a – b)(a^2 + ab + b^2)
This formula can be constructed from expanding (a + b)(a^2 + ab + b2) by using distribution property. The outcome of this expansion is a3 + ab2 + ab2b – b3. Note that the middle terms, ab2 and A2b, cancel one another out, leaving only a3 and b3.
The formula a3-b3 to calculate the differences of cubes is helpful in terms of algebraic simplification as well as factoring. It permits you to factor out expressions that take the form of a difference of cubes making it simpler to solve equations that require such expressions.
A3+b3 Formula – a^3 + b^3 Formula
The formula you’re using, “a3 + b3,” is an amount of cubes. Similar to the differences of cubes formula, this could be incorporated into a binomial product. The formula is like this:
a^3 + b^3 = (a + b)(a^2 – ab + b^2)
This formula can be constructed through expanding (a + b)(a^2 ab + b2) with distribution property. The outcome of the expansion will be a3 + ab2 + a2b + b3. The middle terms, ab2 as well as ab2 cancel one another and leave only ab2 + b3.
This formula, which is derived from the Sum of Cubes can be beneficial in algebraic simplification and factoring. It lets you factor expressions that take the form of cubes as a sum which makes it simpler to solve problems involving these formulas.
(a+b)^3 Formula
The formula you’re talking about, “(a + b)^3,” is the cube of the binomial. It is expandable by applying this binomial theory. This formula can be described as follows:
(a + b)^3 = a^3 + 3a^2b + 3ab^2 + b^3
The formula can be deduced using the binomial theory, that states that for any integer positive n:
(a + b)^n = C(n, 0)a^n + C(n, 1)a^(n-1)b + C(n, 2)a^(n-2)b^2 + … + C(n, n-1)ab^(n-1) + C(n, n)b^n
In the scenario that (a + b)^3 where 3 is n The binomial coefficients are as in the following order:
C(3, 0) = 1 C(3, 1) = 3 C(3, 2) = 3 C(3, 3) = 1
In the event that we substitute these coefficients into the formula we will get:
(a + b)^3 = 1a^3 + 3a^2b + 3ab^2 + 1b^3
By simplifying, we get the extended form of (a + b)^3:
(a + b)^3 = a^3 + 3a^2b + 3ab^2 + b^3
Formula for the binomial cube, or the expanded version of (a + b)^3 is a way to simplify and extend expressions that involve cube binomials.
a3b3 Formula Proof- a3+b3, a3-b3 Formula Solution
The formula a3+b3 is aB3 formula solutions are listed below in step by steps.
The formula a^3 + b^3
Find out more about a3 B3 Formula formula with evidence.
a3 – b3 = (a – b)(a2 + ab + b2)
This can be proven through the use of right-hand side phrase.
(a – b)(a2 + ab + b2) = a(a2 + ab + b2) – b(a2 + ab + b2 )
=(a (a) – b)(a2 + ab ab2) = ab3 + ab2 + ab2 + a2b + ab2 + b3 [by removing out the normal number (a-b) outab2 + a2b = a3
= a3 + a2b – ab2 + ab2Ab2 + b3 [by joining the terms and removing the terms like ab2 and a2b on the right-hand side of the termab2 and a2b in the left hand side
= (a – b)(a2 + ab + b2) = a3 – b3 = L.H.S
a^3 + b^3 formula
The formula you’re discussing is probably one of them.
a^3 + b^3 = (a + b) * (a^2 – ab + b^2)
For proving this equation we can employ algebraic manipulation. Begin with the left-hand portion of the equation.
a^3 + b^3
Now, let’s calculate the cubes’ sum on the left-hand side.
a^3 + b^3 = (a + b) * (a^2 – ab + b^2)
Let’s extend the right-hand part of the equation to determine if we can get the left-hand side.
(a + b) * (a^2 – ab + b^2) = a * (a^2 – ab + b^2) + b * (a^2 – ab + b^2)
Disseminate the terms in each of the parenthesis
A * a2 = a ab * + B* a2 Ab + b * b2
Simplify each word:
a^3 – a^2b + ab^2 + a^2b – ab^2 + b^3
Note that the words “-a^2b + a^2b” and “-ab^2 + ab^2” cancel one another out:
a^3 + b^3
Voila! We’ve found the left part of the equation that is, that the sum of cubes formula:
a^3 + b^3 = (a + b) * (a^2 – ab + b^2)
This formula is an effective tool for manipulating algebraic equations using cubic expressions.
A3 b3 Formula Solutions And Sample Examples
A3 B3 Questions based on formulas and examples are listed below.
Problem: Factorize 27×3 – 64y3
Solution The solution is to write 27×3 and 64y3 as (3x)3 3x – (4y)3.
So, applying the formula:
a3 – b3 = (a – b) (a2 + ab + b2),
We can identify the reasons as follows:
(3x – 4y) (9×2 + 16y2 + 12xy)
Problem: Factorize x3 + 8
Solution: The equation x3 +8 can be written in the form:
3.23 +. Thus, it’s in the form of
a3 + b3.
Therefore, employing the formula:
a3 + b3 = (a + b) (a2 – ab + b2)
We can identify the reasons as follows:
(x + 2) (x2 – x . 2 + 22)
= (x + 2) ( x2 – 2x +4)
Problem: Find 83 – 33
Solution: Applying the formula:
a3 – b3 = (a – b) (a2 + ab + b2),
We get the answer in the form of:
(5) x (82 + 9 + 24) = 485
Problem: Factorize (2x + y)3 – (x + 2y)3
Solution: Since this equation follows the formula A3 – B3,
Thus, applying the formula:
a3 – b3 = (a – b) (a2 + ab + b2),
We can identify the reasons as follows:
(2x + y – x – 2y) [(2x + y)2 + (x + 2y)2 + (2x + y) (x + 2y)]
= (x – y) (7×2 + 7y2 + 13xy)








Leave a Reply